Bound To Stay Bound

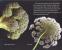
School Library Journal - 03/01/2014 Gr 3–6—The team who explored the Fibonacci sequence in Growing Patterns (Boyds Mills, 2010) returns with a similar book about fractals. Until 1975, there was no name for shapes in nature in which smaller parts looked like the whole shape. Then mathematician Benoit Mandelbrot, who had been thinking about and studying these patterns, named them fractals. Using clear text and outstanding color photographs, Campbell explores the concept of these unusual shapes. Beginning with circles, cones, and cylinders, she leads readers carefully and concisely through examples of fractals such as trees, rivers, mountains, broccoli, lightning, and lungs. The photographs, sometimes highlighting the ever-smaller pieces of a vegetable fractal against a black background, sometimes drawing back to give a aerial view of a geological feature, are crisp and precise and underscore the clear text. The book invites readers to construct a geometric fractal as a hands-on exemplar of the concept. An afterword reveals more of Mandelbrot's background and work, which will be an inspiration to budding scientists/mathematicians.—Marge Loch-Wouters, La Crosse Public Library, WI - Copyright 2014 Publishers Weekly, Library Journal and/or School Library Journal used with permission.

Bulletin for the Center... - 05/01/2014 Campbell, who introduced young audiences to Fibonacci number sequences in Growing Patterns (BCCB 5/10), returns with a similar approach to recognizing fractals in the natural world. She begins with an illustrated discussion of perfect shapes, such as a circle, cone, or cylinder, and demonstrates how objects in nature approximate these shapes. Then she moves toward Benoit Mandelbrot’s observation that natural shapes, although not perfect, often appear in a particular self-similar pattern, or, as Campbell puts it, “Every fractal shape has smaller parts that look like the whole shape,” using a leafless tree and a diagram of complex branching to demonstrate. The remainder of the text and images provide further examples, from broccoli to bronchioles, and a couple of examples of non-fractal patterns, such as pineapple husk and caterpillar markings. The definition of fractals is annoyingly late in arriving, though, and it is buried amid text that makes it easy to miss. Moreover, selected images vary in their efficacy for revealing fractal patterns: it’s far easier to recognize structural similarities in lung airways than in the aerial photo of the Colorado River. A Make Your Own Fractal activity, with simple directions and diagrams for constructing a Sierpinski triangle, goes a long way in providing clarity, and Yale mathematician Michael Frame’s closing notes on Mandelbrot and the practicality of his observations extends the view of fractals beyond natural phenomenon. EB - Copyright 2014 The Board of Trustees of the University of Illinois.

Booklist - 04/01/2014 The creators of Growing Patterns: Fibonacci Numbers in Nature (2010) present another mathematical concept related to patterns and fractals. After introducing classic geometric shapes, the discussion shifts to Mandelbrot’s observations of fractals with a brief explanation (“Every fractal shape has smaller parts that look like the whole shape”) and a series of examples. In the first, a line segment branches into a Y, with each arm branching again and again into ever smaller Y’s, while an adjacent photo shows a bare-branched tree. Many clear color photos illustrate the examples, which include a broccoli crown, a clustered flower head, lightning, and a mountain range. The latter may be hard for children to grasp as an example of fractals, as there’s little clarification and no graphic aid apart from a photo of mountain peaks. The afterword comments on Mandelbrot and some possible applications of his ideas (invisibility cloaks, anyone?). While clearer explanations would have made this a stronger book, this beautifully designed volume is a useful resource and, apparently, the only children’s book devoted to fractals. - Copyright 2014 Booklist.

Loading...